價格:免費
更新日期:2018-08-01
檔案大小:7.9 MB
目前版本:2.5
版本需求:需要 iOS 11.3 或以上版本。與 iPhone、iPad 及 iPod touch 相容。
支援語言:英語
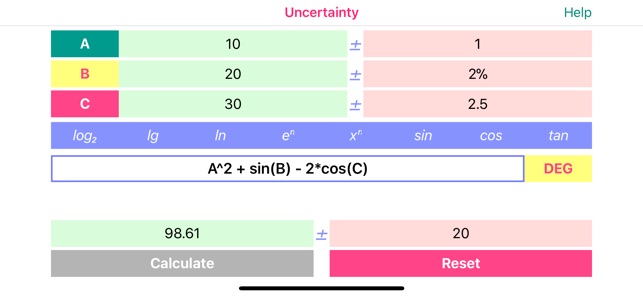
Uncertainty aims to evaluate the error propagation throughout the mathematical expressions comprised of variables of uncertain value.
The application allows input of up to three value-error pairs (A, B and C), that can be used as a parameters in the final expression.
Error values should be provided in absolute (100 +/- 5) or in relative format, the latter should appear in percent format (100 +/- 5%).
The evaluated expression can include numerous functions, that provided by row of the appropriate buttons.
Components and functions can be added directly to expression field by pressing the corresponding buttons. To encompass expression into the preset formula, select the expression and press the corresponding formula button.
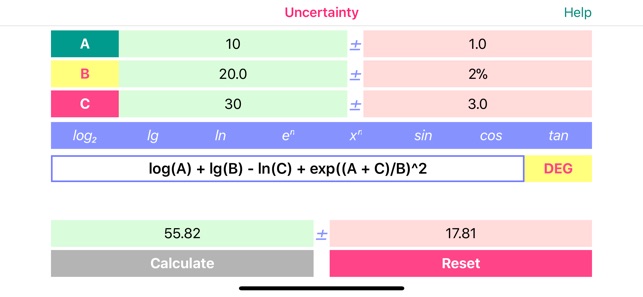
Particular attention should be paid to placing brackets to avoid equation ambiguity.
The application deals with independent, random errors. So if this is a case never use A + A, but rather 2*A and never A*A*A but rather A^3! Obviously, different parameters can be combined in any way (A+B or C *A^2 .. is perfectly fine).
Angles for trigonometric functions can be provided in degrees or radians, and that should be reflected by setting a yellow button to "DEG" or "RAD". Bear in mind the importance of
units in any of calculations!
Implementation Examples:
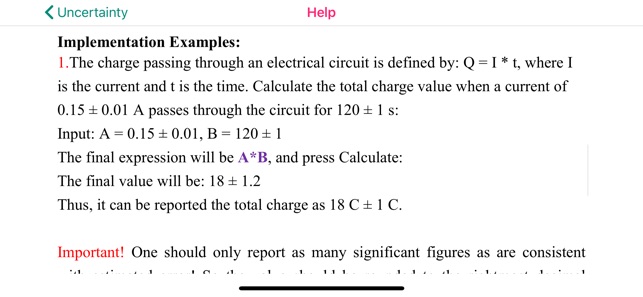
1.The charge passing through an electrical circuit is defined by: Q = I * t, where I is the current and t is the time. Calculate the total charge value when a current of 0.15 ± 0.01 A passes through the circuit for 120 ± 1 s:
Input: A = 0.15 ± 0.01, B = 120 ± 1
The final expression will be A*B, and press Calculate:
The final value will be: 18 ± 1.2
Thus, it can be reported the total charge as 18 C ± 1 C.
Important! One should only report as many significant figures as are consistent with estimated error! So the value should be rounded to the rightmost decimal place at which error applies.
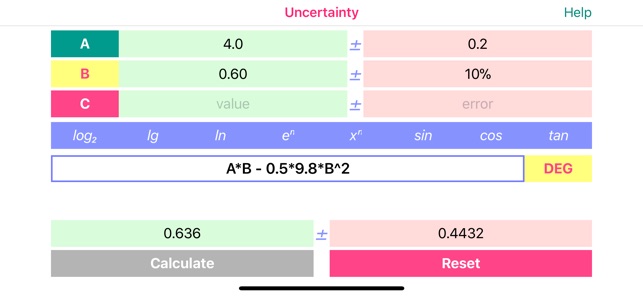
2. A ball is tossed straight up into the air with initial speed vo = (4.0± 0.2) m/s. After a time
t = (0.60 ± 10%) s, the height of the ball is h = vo*t - 0.5gt^2 = 0.636 m (g = 9.80 m/s^2). What is the value and uncertainty of height?
Input: A = vo = 4.0 ± 0.2, and B = t = 0.60 ± 10%, then the final expression should be:
A*B – 0.5*9.8*B^2
The final value will be: 0.6 ± 0.4 (meters).

支援平台:iPhone, iPad
